Volume of Shapes 4
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 6 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- 5
- 6
- Answered
- Review
-
Question 1 of 6
1. Question
Find the volume of the PyramidRound your answer to one decimal place- Volume =Volume = (1326.6, 1324.9) cm3cm3
Hint
Help VideoCorrect
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Volume of a Pyramid
Volume =13×Volume =13×lengthlength××widthwidth××heightheightLabelling the given lengths
length=18length=18width=11width=11height=?height=?b (right triangle)=9b (right triangle)=9 ((1818÷2)÷2)c (right triangle)=22c (right triangle)=22First, we need to find the height perpendicular to its base.Label the sides of the right triangle formed within the pyramidUse the Pythagorean Theorem Formula to solve for aa, which is equal to the heightheighta2a2++b2b2 == c2c2 Pythagoras’ Theorem Formula height2height2++9292 == 222222 Plug in the known lengths height2+81height2+81 == 484484 Evaluate 9292 and 222222 height2height2 == 403403 Subtract 8181 from both sides heightheight == 20.1 cm20.1 cm Take the square root of both sides Next, find the area of the pyramid’s base, which is a rectangleAreaArea == lengthlength××widthwidth Area of a Rectangle == 1818××1111 Plug in the known lengths == 198 cm2198 cm2 Finally, use the formula to find the volumeNote that areaarea==lengthlength××widthwidthVolumeVolume == 13×13×lengthlength××widthwidth××heightheight Volume of a Pyramid == 13×13×198198××20.120.1 Plug in the known lengths == 1326.6 cm31326.6 cm3 The given measurements are in centimetres, so the volume is measured as centimetres cubedVolume=1326.6 cm3Volume=1326.6 cm3 -
Question 2 of 6
2. Question
What is the volume of this cylinder?
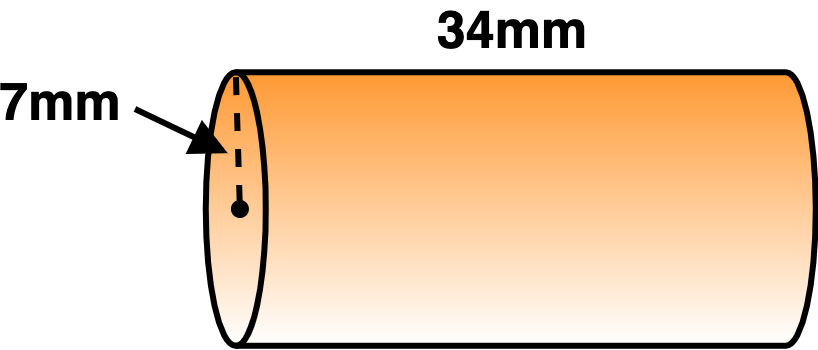 Round your answer to 22 decimal placesUse π≈3.14π≈3.14
Round your answer to 22 decimal placesUse π≈3.14π≈3.14- Volume== (5281.24)mm3mm3
Correct
Keep Going!
Incorrect
Volume of a Cylinder
V=π×radius2×heightV=π×radius2×heightLabelling the given lengths
height=34height=34radius=7radius=7 Use the formula to find the volumeπ≈3.14π≈3.14
Use the formula to find the volumeπ≈3.14π≈3.14VV == π×radius2×heightπ×radius2×height Volume of a cylinder formula == 3.14×72×343.14×72×34 Plug in the known lengths == 3.14×49×343.14×49×34 Simplify == 5,281.24 mm35,281.24 mm3 Rounded to 2 decimal places The given measurements are in millimetres, so the volume is measured as millimetres cubedVolume=5,281.24 mm3=5,281.24 mm3 -
Question 3 of 6
3. Question
What is the volume of this cone?
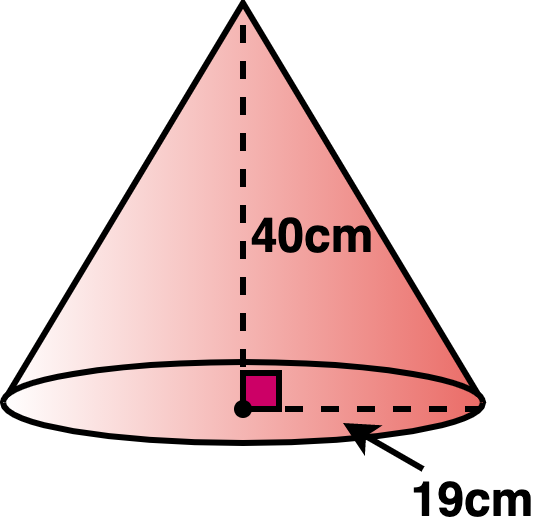 Round your answer to 22 decimal placesUse π≈3.14π≈3.14
Round your answer to 22 decimal placesUse π≈3.14π≈3.14- Volume== (15113.87)cm3cm3
Correct
Keep Going!
Incorrect
Volume of a Cone
V=13×π×radius2×heightV=13×π×radius2×heightLabelling the given lengths
height=40height=40radius=19radius=19 Use the formula to find the volumeπ≈3.14π≈3.14
Use the formula to find the volumeπ≈3.14π≈3.14VV == 13×π×radius2×height13×π×radius2×height Volume of a cone formula == 13×3.14×192×4013×3.14×192×40 Plug in the known lengths == 13×3.14×361×4013×3.14×361×40 Simplify == 15,113.8666715,113.86667 == 15,113.87 cm315,113.87 cm3 Rounded to 2 decimal places The given measurements are in centimetres, so the volume is measured as centimetres cubedVolume=15,113.87 cm3=15,113.87 cm3 -
Question 4 of 6
4. Question
What is the volume of this Rectangular Pyramid?
- Volume== (50)mm3mm3
Correct
Keep Going!
Incorrect
Volume of a Rectangular Pyramid
V=13×length×width×heightV=13×length×width×heightLabelling the given lengths
length=5length=5width=3width=3height=10height=10Use the formula to find the volumeVV == 13×length×width×height13×length×width×height Volume of a Rectangular Pyramid formula == 13×5×3×1013×5×3×10 Plug in the known lengths == 5050 == 50 mm350 mm3 The given measurements are in millimetres, so the volume is measured as millimetres cubedVolume=50 mm3=50 mm3 -
Question 5 of 6
5. Question
Find the volume of the ConeRound your answer to 11 decimal placeUse π=3.141592654π=3.141592654- Volume =Volume = (5305.8, 5303.1, 5307.9) cm3cm3
Hint
Help VideoCorrect
Exceptional!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Volume of a Cone
Volume=13×π×Volume=13×π×radius2radius2××heightheightLabelling the given lengths
radius=?radius=?diameter=23diameter=23height=?height=?c (right triangle)=40c (right triangle)=40First, recall that the radius is equal to half of the diameterradiusradius == 12×12×2323 radiusradius == 11.511.5 Next, we need to find the height perpendicular to its base.Label the sides of the right triangle formed within the pyramidUse the Pythagorean Theorem Formula to solve for aa, which is equal to the heighta2+b2 = c2 Pythagoras’ Theorem Formula height2+11.52 = 402 Plug in the known lengths height2+11.52 = 402 Evaluate 11.52 and 402 height2+132.25 = 1600 Subtract 132.25 from both sides √height2 = √1467.75 Take the square root of both sides height = 38.3112255 cm Finally, use the formula to find the volumeUse π=3.141592654 See π explainedVolume = 13×π×radius2×height Volume of a Cone formula = 13×3.141592654×11.52×38.3112255 Plug in the known lengths = 13×3.141592654×132.25×38.3112255 Simplify = 5305.79349 = 5305.8 cm3 Rounded to one decimal place The given measurements are in centimetres, so the volume is measured as centimetres cubedVolume=5305.8 cm3The answer will depend on which π you use.In this solution we used: π=3.141592654.Using Answer π=3.141592654 5305.8 cm3 π=3.14 5303.1 cm3 π=227 5307.9 cm3 -
Question 6 of 6
6. Question
What is the volume of this hemisphere?
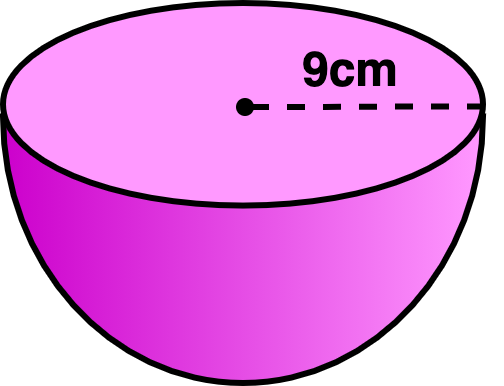 Round your answer to 2 decimal placesUse π≈3.14
Round your answer to 2 decimal placesUse π≈3.14- Volume= (1526.04)cm3
Correct
Keep Going!
Incorrect
Volume of a Hemisphere
V=23×π×radius3Labelling the given lengths
radius=9 Use the formula to find the volumeπ≈3.14
Use the formula to find the volumeπ≈3.14V = 23×π×radius3 Volume of a hemisphere formula = 23×3.14×93 Plug in the known lengths = 23×3.14×729 Simplify = 1,526.04 cm3 Rounded to 2 decimal places The given measurements are in centimetres, so the volume is measured as centimetres cubedVolume=1,526.04 cm3
Quizzes
- Volume of Shapes 1
- Volume of Shapes 2
- Volume of Shapes 3
- Volume of Shapes 4
- Volume of Composite Shapes 1
- Volume of Composite Shapes 2
- Surface Area of Shapes 1
- Surface Area of Shapes 2
- Surface Area of Shapes 3
- Surface Area and Volume Mixed Review 1
- Surface Area and Volume Mixed Review 2
- Surface Area and Volume Mixed Review 3
- Surface Area and Volume Mixed Review 4










