Topics
>
Trigonometry>
Bearings>
Using Bearings to Find Distance>
Using Bearings to Find Distance 2Using Bearings to Find Distance 2
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 4 questions completed
Questions:
- 1
- 2
- 3
- 4
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- Answered
- Review
-
Question 1 of 4
1. Question
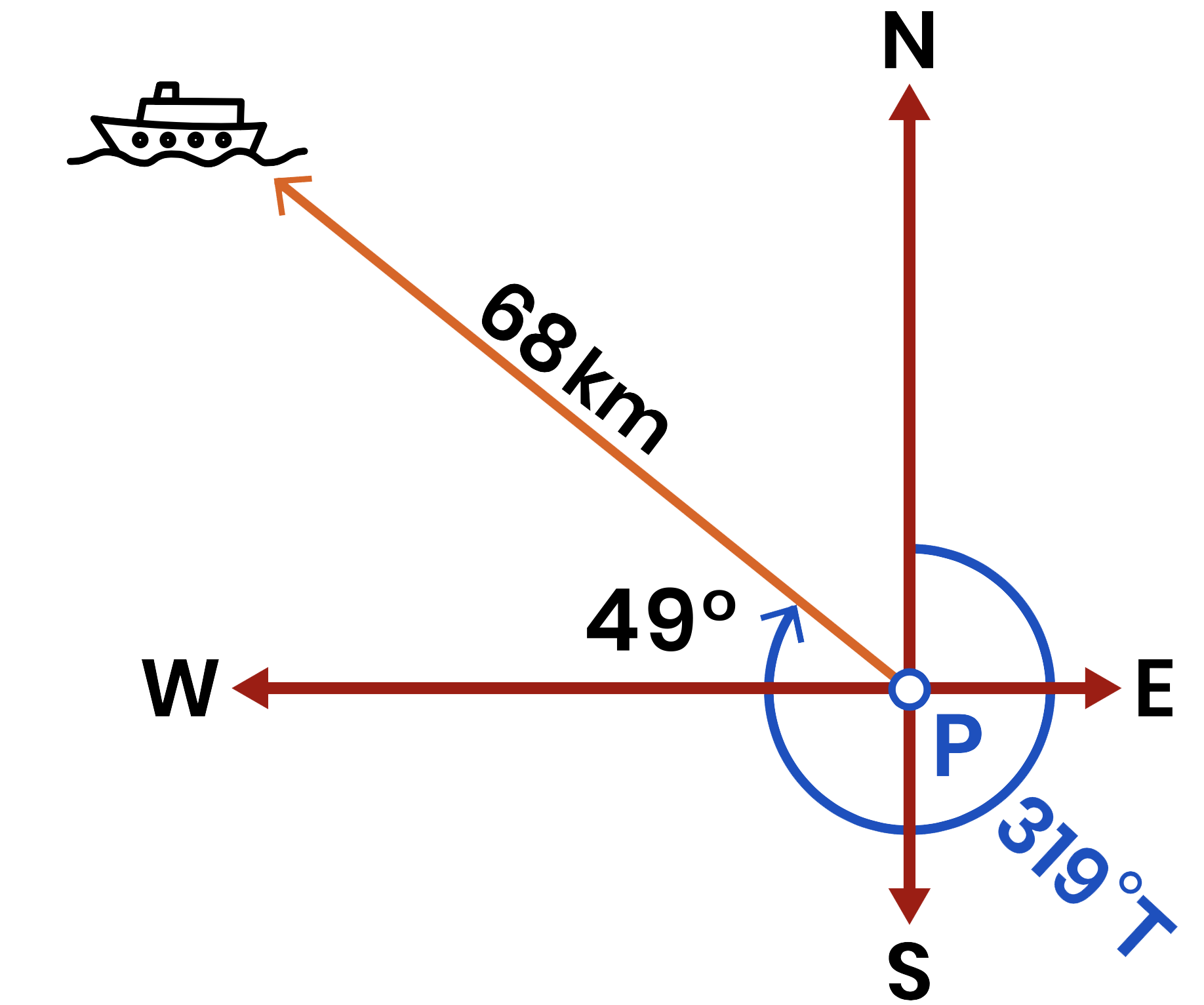
A ship has traveled `68 \text(km)` northwest from point `P` with a true bearing of `319°T`. How far west (`x_w`) has it traveled?
Round your answer to one decimal place- `x_w=` (44.6) `\text(km)`
Hint
Help VideoCorrect
Nice Job!
Incorrect
Sin Ratio
$$sin=\frac{\color{#004ec4}{\text{opposite}}}{\color{#e85e00}{\text{hypotenuse}}}$$Cos Ratio
$$cos=\frac{\color{#00880a}{\text{adjacent}}}{\color{#e85e00}{\text{hypotenuse}}}$$Tan Ratio
$$tan=\frac{\color{#004ec4}{\text{opposite}}}{\color{#00880a}{\text{adjacent}}}$$A true bearing is an angle measured clockwise from the True North around to the required direction.First, find the value of `theta` below.Notice that it is part of the given bearing, which also consists of `3` quadrants from the North line moving clockwise.
To find the value of `theta`, simply subtract the measure of the angles from the three quadrants, `270°`, from the given bearing.`theta` `=` `319°-270°` `=` `49°` To solve for `x_w`, we can use the known values of the hypotenuse and `theta=49°`.
Use `cos` to find the value of `x_w`.`cos49°` `=` $$\frac{\color{#00880A}{x_w}}{\color{#e85e00}{\text{hypotenuse}}}$$ `cos49°` `=` $$\frac{\color{#00880A}{x_w}}{\color{#e85e00}{68}}$$ `cos49°``xx68` `=` `((x_w)/68)``xx68` Multiply both sides by `68` `68cos49°` `=` `x_w` `x_w` `=` `68cos49°` Using your calculator, `68cos49°=44.6`.Therefore, the speedboat is `44.6 \text(km)` to the West.
`x_w=44.6 \text(km)` -
Question 2 of 4
2. Question
A marathon runner runs `25.3 \text(km)` at a true bearing of `129°T`. Find how far east (`x_e`) the runner has traveled from the starting point (`S`).
Round your answer to one decimal place- `x_e=` (19.7) `\text(km)`
Hint
Help VideoCorrect
Fantastic!
Incorrect
Sin Ratio
$$sin=\frac{\color{#004ec4}{\text{opposite}}}{\color{#e85e00}{\text{hypotenuse}}}$$Cos Ratio
$$cos=\frac{\color{#00880a}{\text{adjacent}}}{\color{#e85e00}{\text{hypotenuse}}}$$Tan Ratio
$$tan=\frac{\color{#004ec4}{\text{opposite}}}{\color{#00880a}{\text{adjacent}}}$$A true bearing is an angle measured clockwise from the True North around to the required direction.First, find the value of `theta` below.Notice that it is part of the given bearing, which also consists of a right angle from the North line moving to the East line.
To find the value of `theta`, simply subtract the value of a right angle, `90°`, from the given bearing.`theta` `=` `129°-90°` `=` `39°` To solve for `x_e`, we can use the known values of the hypotenuse and `theta=39°`.
Use `cos` to find the value of `x_e`.`cos39°` `=` $$\frac{\color{#00880A}{x_e}}{\color{#e85e00}{\text{hypotenuse}}}$$ `cos39°` `=` $$\frac{\color{#00880A}{x_e}}{\color{#e85e00}{25.3}}$$ `cos39°``xx25.3` `=` `((x_e)/25.3)``xx25.3` Multiply both sides by `25.3` `25.3cos39°` `=` `x_e` `x_e` `=` `25.3cos39°` Using your calculator, `25.3cos39°=19.7`.Therefore, the runner runs `19.7 \text(km)` to the East.
`x_e=19.7 \text(km)` -
Question 3 of 4
3. Question
A ship sails on a bearing of `200° T` towards `P`. If `P` is `80` nautical miles west from `O`, find how far the ship has sailed `(x)`.
Round your answer to one decimal place- (233.9) `\text(nautical miles)`
Hint
Help VideoCorrect
Correct!
Incorrect
>Sin Ratio
$$sin=\frac{\color{#004ec4}{\text{opposite}}}{\color{#e85e00}{\text{hypotenuse}}}$$Cos Ratio
$$cos=\frac{\color{#00880a}{\text{adjacent}}}{\color{#e85e00}{\text{hypotenuse}}}$$Tan Ratio
$$tan=\frac{\color{#004ec4}{\text{opposite}}}{\color{#00880a}{\text{adjacent}}}$$A true bearing is an angle measured clockwise from the True North around to the required direction.First, find the value of angle `QOP`.Notice that adding angle `QOP` to the given bearing `(200°)` will cover `3` quadrants, which is equal to `270°`
To find the value of angle `QOP`, simply subtract the value of the bearing from `270°`.`/_QOP` `=` `270°-200°` `=` `70°` To solve for `x`, we can use the known values of the line adjacent to angle `QOP`.
Use `cos` to find the value of `x`.`cos70°` `=` $$\frac{\color{#00880A}{\text{adjacent}}}{\color{#e85e00}{x}}$$ `cos70°` `=` $$\frac{\color{#00880A}{80}}{\color{#e85e00}{x}}$$ `cos70°``xxx` `=` `((80)/x)``xxx` Multiply both sides by `x` `xcos70°` `=` `80` `xcos70°``divcos70°` `=` `80``divcos70°` Divide both sides by `cos70°` `x` `=` `(80)/(cos70°)` Using your calculator, `(80)/(cos70°)=233.9`.Therefore, the boat traveled `233.9 \text(nautical miles)` in total.
`233.9 \text(nautical miles)` -
Question 4 of 4
4. Question
Bianca leaves her home and cycles due North for `12` km, then `7` km due West to go to the gym. How far is the gym from her home `(x)`?
Round your answer to one decimal place- (13.9) `\text(km)`
Hint
Help VideoCorrect
Fantastic!
Incorrect
Pythagoras’ Theorem Formula
`a^2``=``b^2``+``c^2``a` is the hypotenuse, and `b` and `c` are the two legsUse the Pythagoras’ Theorem to solve for the distance `(``x``)`.
`a` `=` `x` `b` `=` `12 \text(km)` `c` `=` `7 \text(km)` `a^2` `=` `b^2``+``c^2` `x^2` `=` `12^2``+``7^2` Substitute known values `sqrt(x^2)` `=` `sqrt(144+49)` Get the square root of both sides `x` `=` `sqrt(193)` `x` `=` `13.9 \text(km)` Rounded to one decimal place 
`13.9 \text(km)`
Quizzes
- Compass Bearings and True Bearings 1
- Compass Bearings and True Bearings 2
- Solving for Bearings
- Bearings from Opposite Direction
- Using Bearings to Find Distance 1
- Using Bearings to Find Distance 2
- Using Bearings to Find Distance 3
- Using Bearings and Distances to Find Angles
- Working with Radial Surveys 1
- Working with Radial Surveys 2
- Working with Radial Surveys 3
- Working with Radial Surveys 4