Volume of Shapes 2

Topics

Geometry

Surface Area and Volume

Volume of Shapes

Volume of Shapes 2

Try VividMath Premium to unlock full access

Start Free Trial

Lets see your results!

Points

Score

Time

Retry Quiz

Answered
Review

Question 1 of 8

1. Question
Find the volume of the Parallelogram
- $Volume =$ $\text(Volume )=$ (280) ${cm}^{3}$ $\text(cm)^3$
Hint

Help Video
Correct

Keep Going!
Incorrect
Need Text
Play
Current Time 0:00
/
Duration Time 0:00
Remaining Time -0:00
Stream TypeLIVE
Loaded: 0%
Progress: 0%
0:00
Fullscreen
Video Acceleration:
On
Off

00:00
Mute
Playback Rate
1x
2x
1.5x
1.25x
1x
0.75x
0.5x
Subtitles
subtitles off
Captions
captions off
English
Chapters
Chapters

Volume of a Parallelogram

$Volume =$ $\text(Volume )=$ $base$ $\text(base)$ $\times$ $times$ $height$ $\text(height)$ $\times$ $times$ $depth$ $\text(depth)$

Labelling the given lengths

$base = 7$ $\text(base)=7$

$height = 5$ $\text(height)=5$

$depth = 8$ $\text(depth)=8$

First, find the area of the front face

$Area$ $\text(Area)$ $=$ $=$ $base$ $\text(base)$ $\times$ $times$ $height$ $\text(height)$ Area of a Paralellogram

$=$ $=$ $7$ $7$ $\times$ $times$ $5$ $5$ Plug in the known lengths

$=$ $=$ $35 {cm}^{2}$ $35 \text(cm)^2$

Next, multiply the area by the depth to find the volume

$Volume$ $\text(Volume)$ $=$ $=$ $area$ $\text(area)$ $\times$ $times$ $depth$ $\text(depth)$ Finding the volume

$=$ $=$ $35$ $35$ $\times$ $times$ $8$ $8$ Plug in the known lengths

$=$ $=$ $280 {cm}^{3}$ $280 \text(cm)^3$

The given measurements are in centimetres, so the volume is measured as centimetres cubed

$Volume = 280 {cm}^{3}$ $\text(Volume)=280 \text(cm)^3$
Question 2 of 8

2. Question
What is the volume of this cube?
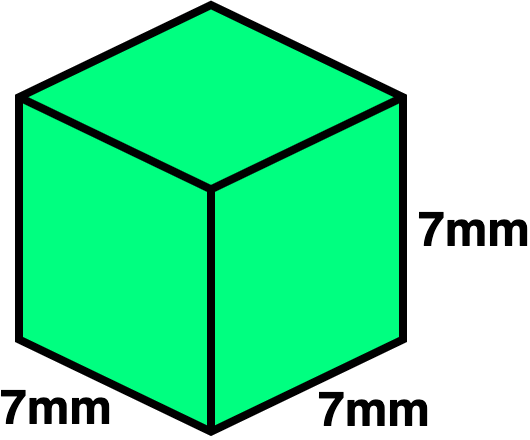
- Volume $=$ $=$ (343) $m m^{3}$ $mm^3$
Correct

Keep Going!

Incorrect

Volume of a Cube

$V = s^{3}$ $V=color(darkviolet)(s)^3$

Labelling the given lengths

$side = 7$ $color(darkviolet)(\text(side)=7)$

Use the formula to find the volume

$V$ $V$ $=$ $=$ $s^{3}$ $color(darkviolet)(s)^3$ Volume of a cube formula

$=$ $=$ $7^{3}$ $color(darkviolet)(7)^3$ Plug in the known lengths

$=$ $=$ $343$ $343$ Simplify

$=$ $=$ $343 m m^{3}$ $343 \ mm^3$

The given measurements are in millimetres, so the volume is measured as millimetres cubed

Volume $= 343 m m^{3}$ $=343 \ mm^3$

Question 3 of 8

What is the volume of this Rectangular Prism?

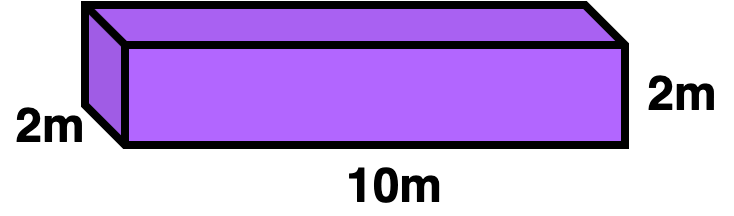
Volume $=$ $=$ (40) $m^{3}$ $m^3$

Incorrect

Volume of a Rectangular Prism

V = height \times width \times depth

$V=color(royalblue)(\text(height)) xx color(darkviolet)(\text(width))xx color(green)(\text(depth))$

Labelling the given lengths

height = 2

$color(royalblue)(\text(height)=2)$

width = 10

$color(darkviolet)(\text(width)=10)$

depth = 2

$color(green)(\text(depth)=2)$

Use the formula to find the volume

$V$ $V$	$=$ $=$	$height \times width \times depth$ $color(royalblue)(\text(height)) xx color(darkviolet)(\text(width))xx color(green)(\text(depth))$	Volume of a Rectangular Prism formula

	$=$ $=$	$2 \times 10 \times 2$ $color(royalblue)(\text(2)) xx color(darkviolet)(\text(10))xx color(green)(\text(2))$	Plug in the known lengths

	$=$ $=$	$40$ $40$
	$=$ $=$	$40 m^{3}$ $40 \ m^3$

The given measurements are in metres, so the volume is measured as metres cubed

Volume

= 40 m^{3}

$=40 \ m^3$

Question 4 of 8

Find the volume of the Prism

$Volume =$ $\text(Volume )=$ (1092) $m^{3}$ $\text(m)^3$

Incorrect

Need Text

Play

Remaining Time -0:00

Stream TypeLIVE

Loaded: 0%

Progress: 0%

0:00

Fullscreen

00:00

Mute

Volume of a Trapezoidal Prism

Volume = \frac{1}{2} \times

$\text(Volume )=1/2 times$ $height$ $\text(height)$

\times (

$times ($ ${base}_{1}$ $\text(base)_1$

+

$+$ ${base}_{2}$ $\text(base)_2$

)

$)$

\times

$times$ $depth$ $\text(depth)$

Labelling the given lengths

height = 12

$\text(height)=12$

{base}_{1} = 8

$\text(base)_1=8$

{base}_{2} = 18

$\text(base)_2=18$

depth = 7

$\text(depth)=7$

First, find the area of the front face

$Area$ $\text(Area)$	$=$ $=$	$\frac{1}{2} \times$ $1/2 times$ $height$ $\text(height)$ $\times ($ $times ($ ${base}_{1}$ $\text(base)_1$ $+$ $+$ ${base}_{2}$ $\text(base)_2$ $)$ $)$	Area of a Trapezoid

	$=$ $=$	$\frac{1}{2} \times$ $1/2 times$ $12$ $12$ $\times ($ $times ($ $8$ $8$ $+$ $+$ $18$ $18$ $)$ $)$	Plug in the known lengths

	$=$ $=$	$156 m^{2}$ $156 \text(m)^2$

Next, multiply the area by the depth to find the volume

$Volume$ $\text(Volume)$	$=$ $=$	$area$ $\text(area)$ $\times$ $times$ $depth$ $\text(depth)$	Finding the volume
	$=$ $=$	$156$ $156$ $\times$ $times$ $7$ $7$	Plug in the known lengths
	$=$ $=$	$1092 m^{3}$ $1092 \text(m)^3$

The given measurements are in metres, so the volume is measured as metres cubed

Volume = 1092 m^{3}

$\text(Volume)=1092 \text(m)^3$

Question 5 of 8

What is the volume of this Triangular Prism?

Volume $=$ $=$ (2002) $m^{3}$ $m^3$

Incorrect

Volume of a Triangular Prism

V = \frac{1}{2} \times base \times height \times depth

$V=1/2 xx color(darkviolet)(\text(base)) xx color(royalblue)(\text(height)) xx color(green)(\text(depth))$

Labelling the given lengths

base = 14

$color(darkviolet)(\text(base)=14)$

height = 13

$color(royalblue)(\text(height)=13)$

depth = 22

$color(green)(\text(depth)=22)$

Use the formula to find the volume

$V$ $V$	$=$ $=$	$\frac{1}{2} \times base \times height \times depth$ $1/2 xx color(darkviolet)(\text(base)) xx color(royalblue)(\text(height)) xx color(green)(\text(depth))$	Volume of a Triangular Prism formula

	$=$ $=$	$\frac{1}{2} \times 14 \times 13 \times 22$ $1/2 xx color(darkviolet)(\text(14))xx color(royalblue)(\text(13)) xx color(green)(\text(22))$	Plug in the known lengths

	$=$ $=$	$2, 002$ $2,002$
	$=$ $=$	$2, 002 m^{3}$ $2,002 \ m^3$

The given measurements are in metres, so the volume is measured as metres cubed

Volume

= 2, 002 m^{3}

$=2,002 \ m^3$

Question 6 of 8

Find the volume of the Cylinder

Round your answer to

1

$1$ decimal place

Use

π = 3.141592654

$pi=3.141592654$

$Volume =$ $\text(Volume )=$ (415122.2, 414911.8, 415289.3) $m^{3}$ $\text(m)^3$

Incorrect

Need Text

Play

Remaining Time -0:00

Stream TypeLIVE

Loaded: 0%

Progress: 0%

0:00

Fullscreen

00:00

Mute

Volume of a Cylinder

Volume = π \times

$\text(Volume)=pi times$ ${radius}^{2}$ $\text(radius)^2$

\times

$times$ $height$ $\text(height)$

Labelling the given lengths

radius = ?

$\text(radius)=?$

diameter = 155

$\text(diameter)=155$

height = 22

$\text(height)=22$

Recall that the radius is equal to half of the diameter

$radius$ $\text(radius)$	$=$ $=$	$\frac{1}{2} \times$ $1/2 times$ $155$ $155$

$radius$ $\text(radius)$	$=$ $=$	$77.5$ $77.5$

Now we can use the formula to find the volume

Use

π = 3.141592654

$pi=3.141592654$ See

π

$pi$ explained

$Volume$ $\text(Volume)$	$=$ $=$	$π \times$ $pi times$ ${radius}^{2}$ $\text(radius)^2$ $\times$ $times$ $height$ $\text(height)$	Volume of a Cylinder formula
	$=$ $=$	$3.141592654 \times$ $3.141592654 times$ ${77.5}^{2}$ $77.5^2$ $\times$ $times$ $22$ $22$	Plug in the known lengths
	$=$ $=$	$3.141592654 \times 6006.25 \times 22$ $3.141592654 times 6006.25 times 22$	Simplify
	$=$ $=$	$415122.1993$ $415122.1993$
	$=$ $=$	$415122.2 m^{3}$ $415122.2 \text(m)^3$	Rounded to one decimal place

The given measurements are in metres, so the volume is measured as metres cubed

Volume = 415122.2 m^{3}

$\text(Volume)=415122.2 \text(m)^3$

The answer will depend on which

π

$pi$ you use.

In this solution we used:

π = 3.141592654

$pi=3.141592654$ .

Using	Answer
$π = 3.141592654$ $pi=3.141592654$	$415122.2 m^{3}$ $415122.2 m^3$
$π = 3.14$ $pi=3.14$	$414911.8 m^{3}$ $414911.8 m^3$
$π = \frac{22}{7}$ $pi=(22)/(7)$	$415289.3 m^{3}$ $415289.3 m^3$

Question 7 of 8

Find the volume of the Sphere

Round your answer to the nearest whole number

Use

π = 3.141592654

$pi=3.141592654$

$Volume =$ $\text(Volume )=$ (17157, 17149, 17164) ${cm}^{3}$ $\text(cm)^3$

Incorrect

Need Text

Play

Remaining Time -0:00

Stream TypeLIVE

Loaded: 0%

Progress: 0%

0:00

Fullscreen

00:00

Mute

Volume of a Sphere

Volume = \frac{4}{3} \times π \times

$\text(Volume)=4/3 times pi times$ ${radius}^{3}$ $\text(radius)^3$

Labelling the given lengths

radius = 16

$\text(radius)=16$

Use the formula to find the volume

Use

π = 3.141592654

$pi=3.141592654$ See

π

$pi$ explained

$Volume$ $\text(Volume)$	$=$ $=$	$\frac{4}{3} \times π \times$ $4/3 times pi times$ ${radius}^{3}$ $\text(radius)^3$	Volume of a Sphere formula

	$=$ $=$	$\frac{4}{3} \times 3.141592654 \times$ $4/3 times 3.141592654 times$ $16^{3}$ $16^3$	Plug in the known lengths

	$=$ $=$	$\frac{4}{3} \times 3.141592654 \times 4096$ $4/3 times 3.141592654 times 4096$	Simplify

	$=$ $=$	$17157.28468$ $17157.28468$
	$=$ $=$	$17157 {cm}^{3}$ $17157 \text(cm)^3$	Rounded to a whole number

The given measurements are in centimetres, so the volume is measured as centimetres cubed

Volume = 17157 {cm}^{3}

$\text(Volume)=17157 \text(cm)^3$

The answer will depend on which

π

$pi$ you use.

In this solution we used:

π = 3.141592654

$pi=3.141592654$ .

Using	Answer
$π = 3.141592654$ $pi=3.141592654$	$17157 c m^{3}$ $17157 cm^3$
$π = 3.14$ $pi=3.14$	$17149 c m^{3}$ $17149 cm^3$
$π = \frac{22}{7}$ $pi=(22)/(7)$	$17164 c m^{3}$ $17164 cm^3$

Question 8 of 8

Find the volume of the Hemisphere

Round your answer to

1

$1$ decimal place

Use

π = 3.141592654

$pi=3.141592654$

$Volume =$ $\text(Volume )=$ (9408.3, 9403.5, 9412.1) ${cm}^{3}$ $\text(cm)^3$

Incorrect

Need Text

Play

Remaining Time -0:00

Stream TypeLIVE

Loaded: 0%

Progress: 0%

0:00

Fullscreen

00:00

Mute

Volume of a Hemisphere

Volume = \frac{1}{2} \times \frac{4}{3} \times π \times

$\text(Volume)=1/2 times 4/3 times pi times$ ${radius}^{3}$ $\text(radius)^3$

Labelling the given lengths

radius = ?

$\text(radius)=?$

diameter = 33

$\text(diameter)=33$

Recall that the radius is equal to half of the diameter

$radius$ $\text(radius)$	$=$ $=$	$\frac{1}{2} \times$ $1/2 times$ $33$ $33$

$radius$ $\text(radius)$	$=$ $=$	$16.5$ $16.5$

Now we can use the formula to find the volume

Use

π = 3.141592654

$pi=3.141592654$ See

π

$pi$ explained

$Volume$ $\text(Volume)$	$=$ $=$	$\frac{1}{2} \times \frac{4}{3} \times π \times$ $1/2 times 4/3 times pi times$ ${radius}^{3}$ $\text(radius)^3$	Volume of a Hemisphere formula

	$=$ $=$	$\frac{1}{2} \times \frac{4}{3} \times 3.141592654 \times$ $1/2 times 4/3 times 3.141592654 times$ ${16.5}^{3}$ $16.5^3$	Plug in the known lengths

	$=$ $=$	$\frac{1}{2} \times \frac{4}{3} \times 3.141592654 \times 4492.125$ $1/2 times 4/3 times 3.141592654 times 4492.125$	Simplify

	$=$ $=$	$9408.28459$ $9408.28459$
	$=$ $=$	$9408.3 {cm}^{3}$ $9408.3 \text(cm)^3$	Rounded to one decimal place

The given measurements are in centimetres, so the volume is measured as centimetres cubed

Volume = 9408.3 {cm}^{3}

$\text(Volume)=9408.3 \text(cm)^3$

The answer will depend on which

π

$pi$ you use.

In this solution we used:

π = 3.141592654

$pi=3.141592654$ .

Using	Answer
$π = 3.141592654$ $pi=3.141592654$	$9408.3 c m^{3}$ $9408.3 cm^3$
$π = 3.14$ $pi=3.14$	$9403.5 c m^{3}$ $9403.5 cm^3$
$π = \frac{22}{7}$ $pi=(22)/(7)$	$9412.1 c m^{3}$ $9412.1 cm^3$

$Area$ $\text(Area)$	$=$ $=$	$base$ $\text(base)$ $\times$ $times$ $height$ $\text(height)$	Area of a Paralellogram
	$=$ $=$	$7$ $7$ $\times$ $times$ $5$ $5$	Plug in the known lengths
	$=$ $=$	$35 {cm}^{2}$ $35 \text(cm)^2$

$V$ $V$	$=$ $=$	$s^{3}$ $color(darkviolet)(s)^3$	Volume of a cube formula

	$=$ $=$	$7^{3}$ $color(darkviolet)(7)^3$	Plug in the known lengths

	$=$ $=$	$343$ $343$	Simplify

	$=$ $=$	$343 m m^{3}$ $343 \ mm^3$

Volume of Shapes 2

Try VividMath Premium to unlock full access

Quiz summary

Information

1. Question

Hint

Keep Going!

Volume of a Parallelogram

Labelling the given lengths

2. Question

Keep Going!

Volume of a Cube

Labelling the given lengths

3. Question

Keep Going!

Volume of a Rectangular Prism

Labelling the given lengths

4. Question

Hint

Well Done!

Volume of a Trapezoidal Prism

Labelling the given lengths

5. Question

Keep Going!

Volume of a Triangular Prism

Labelling the given lengths

6. Question

Hint

Fantastic!

Volume of a Cylinder

Labelling the given lengths

7. Question

Hint

Nice Job!

Volume of a Sphere

Labelling the given lengths

8. Question

Hint

Fantastic!

Volume of a Hemisphere

Labelling the given lengths

Quizzes